From the name itself, a unit circle is a circle with a unit radius. Circles are closed geometric figures without sides or angles. A unit circle has all of the properties of a circle, and its equation is also derived from the equation of a circle. Furthermore, a unit circle is useful for determining the standard angle values of all trigonometric ratios.
We will learn the equation of the unit circle, and we will see how to represent each point on the circumference of the unit circle by using trigonometric ratios of cosθ and sinθ.
Table of Contents
What is a Unit Circle?
Circles with radii of one unit are called unit circles. A unit circle is generally represented in the cartesian coordinate system. The unit circle is algebraically represented by the second-degree equation with two variables x and y. In trigonometry, the unit circle is useful in finding the values of the trigonometric ratios sine, cosine, and tangent.
Unit Circle Definition
As the locus of a point that is one unit away from a fixed point, it is commonly referred to as a unit circle.
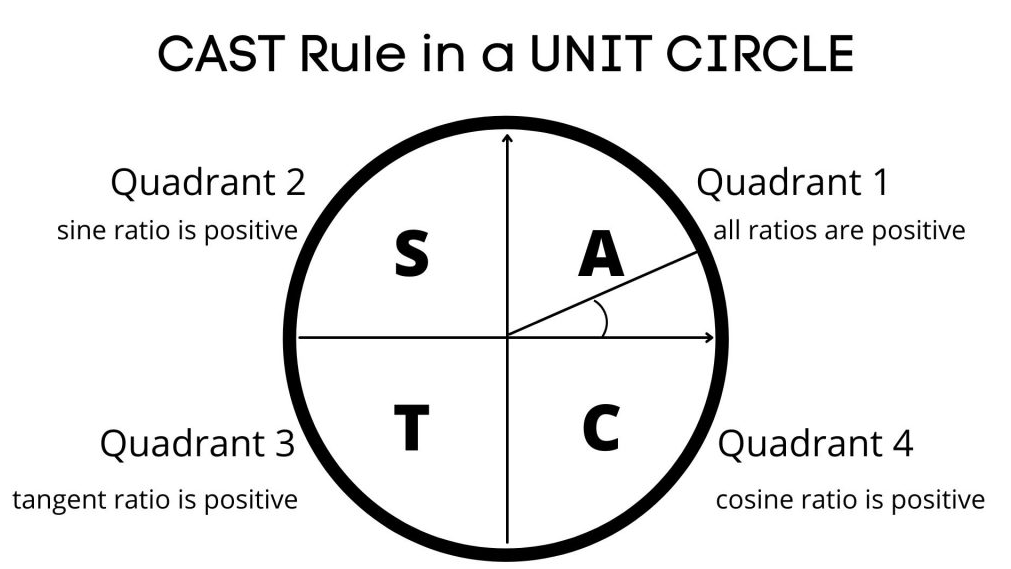
Equation of a Unit Circle
The general equation of a circle is (x – a)2 + (y – b)2 = r2, which describes a circle having the center (a, b) and the radius r. This equation can be simplified to represent the equation of a unit circle. A unit circle is made with its center at the point(0, 0), which is the origin of the coordinate axes. and a radius of 1 unit. Hence the equation of the unit circle is (x – 0)2 + (y – 0)2 = 12. A simplified version of this equation is shown below.
Equation of a Unit Circle: x2 + y2 = 1
The center of the unit circle lies at (0,0) and the radius is 1 unit. All the points in the circle and in the quadrants, according to the above equation, are satisfied.
Finding Trigonometric Functions Using a Unit Circle
The trigonometric functions sine, cosine, and tangent can be calculated using a unit circle. In order to understand trigonometric functions, let us apply the Pythagoras theorem to a unit circle. Consider a right triangle placed within a unit circle in the cartesian coordinate plane. The circumference of the circle is the hypotenuse of the triangle. The radius vector forms an angle θ with the positive x-axis and the coordinates of the endpoint of the radius vector is (x, y). Right triangles have a base and an altitude, which are represented by the values of x and y. We now have a right angle triangle that has sides 1, x, and y. We can find the values of the trigonometric ratio by applying this in trigonometry:
- sinθ = Altitude/Hypoteuse = y/1
- cosθ = Base/Hypotenuse = x/1
We now have sinθ = y, cosθ = x, and using this we now have tanθ = y/x. In the same way, we can calculate the values of the other trigonometric ratios by using the right-angled triangle within the unit circle. Also by changing the θ values we can obtain the greatest values of these trigonometric ratios.
Unit Circle with Sin Cos and Tan
Any point on the unit circle has coordinates(x, y), which are equivalent to the trigonometric identities of (cosθ, sinθ). For any values of θ made by the radius line with the positive x-axis, the coordinates of the endpoint of the radius express the cosine and the sine of the θ values. Here we have cosθ = x, and sinθ = y, and these values are helpful to measure the other trigonometric ratio values. Applying this further we have tanθ = sinθ/cosθ or tanθ = y/x.
Another major point to be understood is that the sinθ and cosθ values always lie between 1 and -1, and the radius value is 1, and it has a value of -1 on the negative x-axis. The entire circle represents a complete angle of 360º and the four quadrant lines of the circle make angles of 90º, 180º, 270º, 360º(0º). At 90º and at 270º the cosθ value is equal to 0 and hence the tan values at these angles are undefined.
Example: Find the value of tan 45º using sin and cos values from the unit circle.
Solution:
We know that tan 45° = sin 45°/cos 45°
Using the unit circle chart:
sin 45° = 1/√2
cos 45° = 1/√2
Therefore, tan 45° = sin 45°/cos 45°
= (1/√2)/(1/√2)
= 1
Answer: Therefore, tan 45° = 1
Unit Circle Chart in Radians
The unit circle represents a complete angle of 2π radians. And the unit circle is divided into four quadrants at angles of π/2, π. 3π/2, and 2π respectively. Further within the first quadrant at the angles of 0, π/6, π/4, π/3, π/2 are the standard values, which are applicable to the trigonometric ratios. The points on the unit circle represent the standard angles of cosine and sine ratios. Observe closely the figure below, where the values are repeated across the four quadrants, but with a change in sign. It is caused by the reference x-axis and y-axis, which are positive on one side and negative on the other side of the origin. In general, we can now find the trigonometric ratio values of standard angles by using the unit circle’s four quadrants.
Unit Circle and Trigonometric Identities
You can also use the unit circle identities of sine, cosecant, and tangent to obtain other trigonometric identities, such as cotangent, secant, and cosecant. These identities are the reciprocal of sine, cosine, and tangent, respectively, for a unit circle. Further, we can obtain the value of tanθ by dividing sinθ with cosθ, and we can obtain the value of cotθ by dividing cosθ with sinθ.
Right triangles placed in a unit circle in the cartesian coordinate plane with hypotenuse, base, and altitude measuring 1, x, y units respectively, have the unit circle identities as follows:
- sinθ = y/1
- cosθ = x/1
- tanθ = sinθ/cosθ = y/x
- sec(θ = 1/x
- csc(θ) = 1/y
- cot(θ) = cosθ/sinθ = x/y
Unit Circle Pythagorean Identities
It is easy to understand and prove the three Pythagorean identities of trigonometric ratios with the unit circle. Pythagoras’ theorem states that, in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. The three Pythagorean identities in trigonometry are listed below.
- sin2θ + cos2θ = 1
- 1 + tan2θ = sec2θ
- 1 + cot2θ = cosec2θ
Our goal is to prove the first identification with the Pythagorean theorem here. Consider x and y as the legs of a right-angled triangle with a hypotenuse of 1 unit. Applying Pythagoras theorem we have x2 + y2 = 1 which describes the equation of a unit circle. Also in a unit circle, we have, x = cosθ, and y = sinθ, and using this in the above statement of the Pythagoras theorem, we have, cos2θ + sin2θ = 1. By using the Pythagoras theorem, we have successfully proven the first identity. The two other Pythagorean identities can be verified further inside the unit circle.
Unit Circle and Trigonometric Values
Using a unit circle, we can calculate the various trigonometric identities and their principal angle values. In the unit circle, cosine is the x-coordinate and sine is the y-coordinate. Let us now see their respective values for θ = 0°, and θ = 90º.
For θ = 0°, the x-coordinate is 1 and the y-coordinate is 0. Therefore, we have cos0º = 1, and sin0º = 0. Let us look at another angle of 90º. Here the value of cos90º = 1, and sin90º = 1. Moreover, let us use this unit circle and find the important trigonometric function values of θ such as 30º, 45º, 60º. Also, we can also contain these θ values in radians. We know that 360° = 2π radians. The angular measures can now be converted to radian measures and expressed in radians.
