The factor theorem establishes a relationship between factors and zeros of a polynomial in algebraic mathematics. As an application of the polynomial remainder theorem, the Factor theorem is unique.
Thus the factor theorem states that a polynomial has a factor if and only if: The polynomial x – M is a polynomial f(x) if and only if f (M) = 0. There is a strong connection between the factor theorem and the remainder theorem; therefore, do not confuse both. It is, however, necessary to explore the remainder theorem in order to uncover the functionality of the actor theorem.
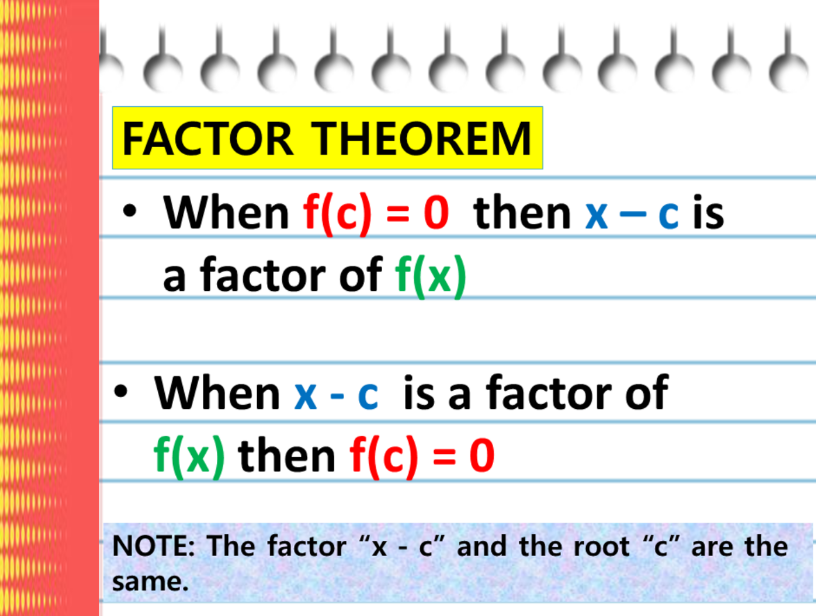
Table of Contents
What is a Factor?
You now know about the remainder theorem. We also need to understand what a “factor” is through our learning of the factor theorem. Knowing precisely what a “factor” is vital to better understand the factor theorem, in fact, to all mathematics concepts. You will hear it time and time again as you proceed with your studies.
Concerning division, a factor is an expression that, when a different expression is divided by this factor, the remainder is equal to zero (0). As a simple example, consider the following: 5 is a factor of 20 because dividing 20 by 5 results in the whole number 4 without a remainder. In the same way, 3 is not a factor of 20 because when we divide 20 by 3, we get 6,67, which is not a whole number.
Factor Theorem Use to Find Polynomial Factors
Factor Theorem is applied to factor polynomials if the remainder in the synthetic division equals zero (0). This proves that the root is undoubtedly a root, and hence the level of “x minus (-) the number” is a factor.
In order to understand the Factor Theorem in-depth and what it means, you must thoroughly comprehend the Remainder Theorem and what factors are first.
Remainder Theorem
It’s essential for algebra students to understand the remainder theorem as well as the factor theorem since they are intricately related. It is true that the former cannot exist without the latter, and the reverse is also true. Having said that, let’s look at the Remainder Theorem.
By the principle of Remainder Theorem:
- Divide any polynomial by (x – M) and the remainder will equal f(c).
The usefulness of Remainder Theorem
The remainder theorem is particularly useful because it significantly decreases the amount of work and calculation we would do to solve such mathematical problems/equations.
Without this theorem, we would be forced to use long division and synthetic division to solve the remainder, which is both difficult and time-consuming. In addition to the visual proof, an analysis of the theories behind the remainder theorem is also very helpful.
Fun Facts
- The factor theorem has a numerical value of 3 based upon the Chaldean Numerology and Pythagorean Numerology.
- Factor theorem assures that an element (x – M) for each root is r.
- Factor theorem does not state that there is only one factor for each root. For this fact, it is pretty easy to create polynomials with arbitrary repetitions of the same root & the same factor
- Factor theorem is helpful as it postulates that factoring a polynomial corresponds to finding roots.
What is the Factor Theorem?
In its most basic terms, the factor theorem is just a particular case of the remainder theorem. In the remainder theorem, if we divide a polynomial f(x) by (x-c), the remainder equals f(c). The factor theorem uses the same concept to prove the following:
According to the Factor Theorem:
In any polynomial division, we must divide by the factor of (x – c), and therefore the remainder resulting from that division is simply zero.
Therefore, this theorem states that if the remainder of a division like those described above equals zero, (x – c) must be a factor. In such a case, if the remainder is not zero, then (x – c) is not a factor.
Solved Examples
Problem 1:
Example: For a curve that crosses the x-axis at 3 points, one is at 2. What is the factor of 2×3−x2−7x+2?
Solution1:
The polynomial for the equation is degree 3 and could be all easy to solve. So let us arrange it first:
We can easily plot:
F (2) = 2 (2)3− (2)2− 7 (2) +2
This brings us:-
= 16−4−14+2
=12 (-14) +2
= -2 + 2
= 0
Thus! F (2) =0, which means we have found a factor and a root
In other words, (x-2) should be a factor of 2×3−x2−7x+2
Problem 2:
Calculate the factor of the polynomial below based on x + 1.
i). 3 x 4 + x 3 – x2 + 3x + 2
Solution 2:
Using the factor theorem,
Let f(x) = 3×4 + x3 – x2 + 3x + 2
That brings to us:-
f (–1) = 3 (–1) 4 + (–1) 3 – (–1)2 +3 (–1) + 2
= 3(1) + (–1) – 1 – 3 + 2 = 0
As a result, we conclude that (x + 1) is a factor of f (x)
