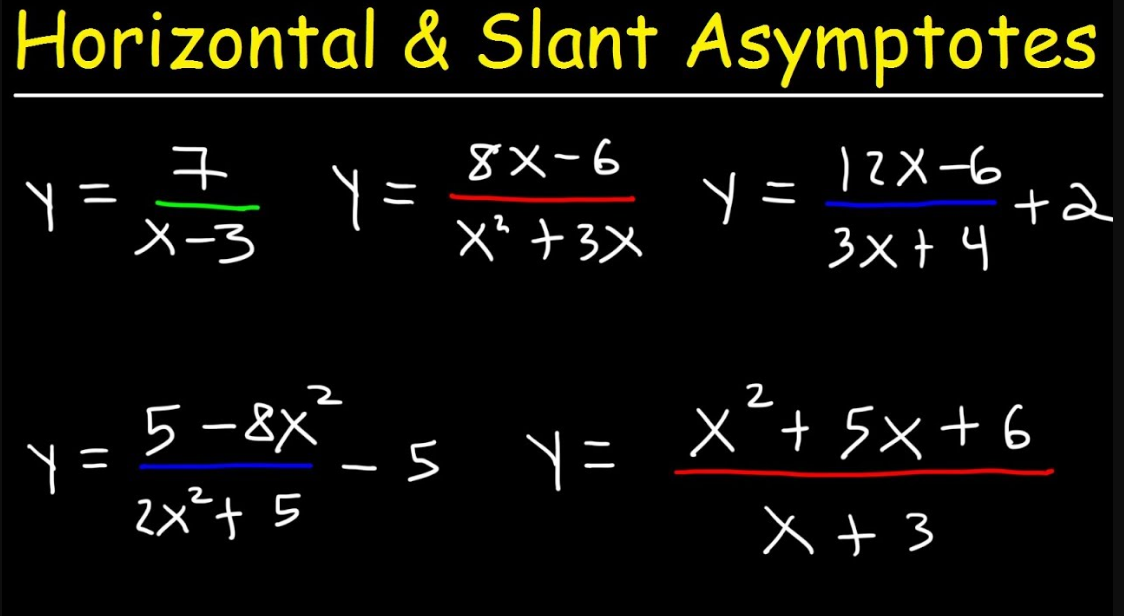
When looking at the numerator and denominator degrees of a rational function, one can find its horizontal asymptotes.
Explanation:
- The horizontal asymptote at y = 0 occurs if the degree of the numerator is less than that of the denominator.
- There is no horizontal asymptote if the degree of the numerator is greater than the degree of the denominator by one.
Another way of finding a horizontal asymptote is by dividing N(x) by D(x). The equation of a horizontal asymptote is y = this constant if the resultant quotient is constant.

Table of Contents
What is a horizontal asymptote?
A horizontal asymptote is a y-value that a function approaches but does not reach on a graph. Here is a simple graphical example where the graphed function comes but never quite matches, y=0. The graph won’t reach zero no matter how far you zoom out. I should however point out that horizontal asymptotes may only appear in one direction and maybe crossed at small values of x. For large values and the trend of a function as x goes towards positive or negative infinity, they will appear.
In order to find horizontal asymptotes, we can write the function as “y=.” You can expect to find horizontal asymptotes when plotting a rational function. As x gets really positive or really negative, the graph of the function grows further and further away from a particular value but never actually reaches that value.
To Find Horizontal Asymptotes:
1) Put equations or functions in y= form.
2) Multiply the factors in the numerator and denominator (expand them).
3) Subtract everything from the numerator and denominator except for the most prominent exponents of x. These are the “dominant” terms.
FAQs
How do you find the horizontal asymptotes?
Another way of finding a horizontal asymptote of a rational function is: Divide N(x) by D(x). In the case of a constant quotient, y = this constant is an equation for a horizontal asymptote.
What are the rules for horizontal asymptotes?
- Y = 0 or the x-axis is the horizontal asymptote when n is less than m.
- The horizontal asymptote is equal to y = a/b when n is equal to m.
- When n is more significant than m, there is no horizontal asymptote.
What are the three types of horizontal asymptotes?
The degree of the numerator exceeds the degree of the denominator by one: no horizontal asymptote; slant asymptote. A horizontal asymptote is reached at a ratio of leading coefficients when the degree of the numerator equals the degree of the denominator.
What is the horizontal asymptote of a function?
Using the denominator degrees and numerator degrees, one can determine a rational function’s horizontal asymptote. The numerator has a degree that is less than the denominator: horizontal asymptote at 0 degrees. Numerator’s degree exceeds that of denominator’s by one: horizontal asymptote absent; slant asymptote present.