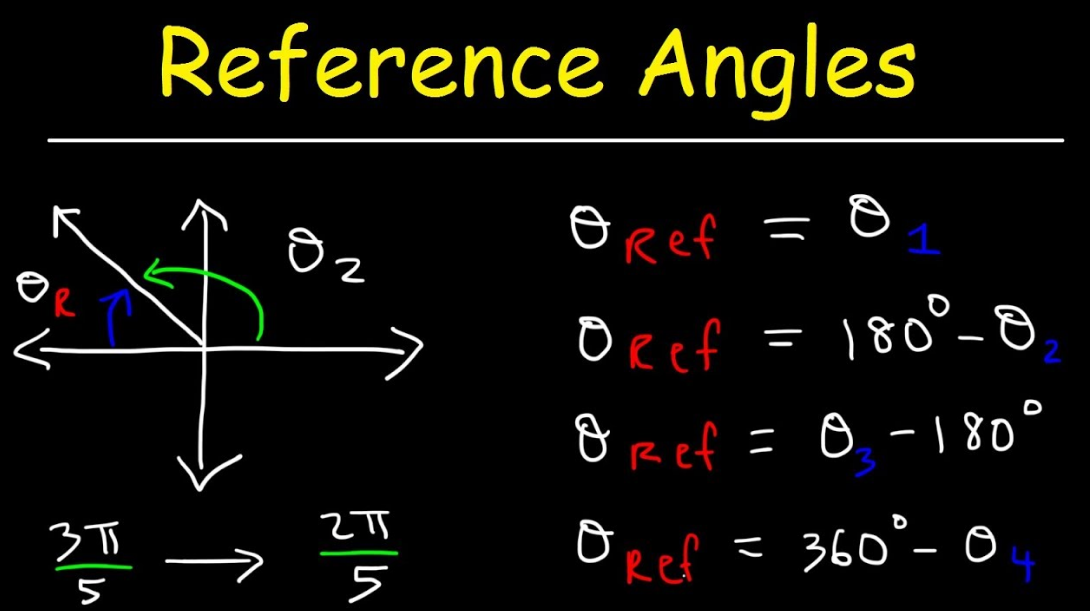
In math, a reference angle is usually an acute angle enclosed between the terminal arm and the x-axis. It is constantly positive and less than or equal to 90 degrees. Let us read more about the reference angle in this article.
Table of Contents
Reference Angle Definition
The reference angle is the minutest possible angle made by the terminal side of the given angle with the x-axis. It is always an acute angle (except when it is precisely 90 degrees). A reference angle is continually positive irrespective of which side of the axis it is falling.
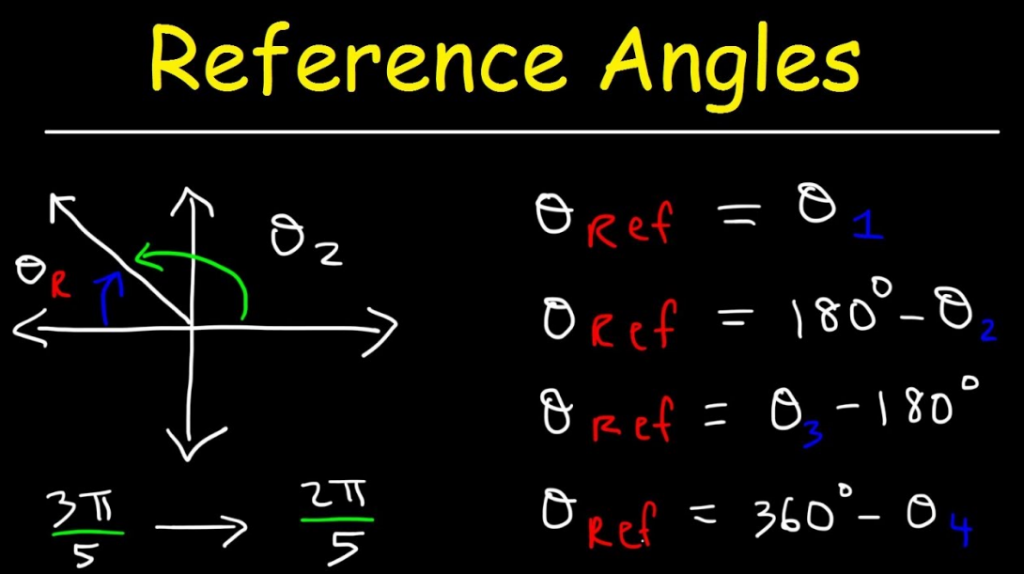
How to Draw Reference Angle?
To represent the reference angle for an angle, identify its terminal side and see by what angle the terminal side is close to the x-axis. The reference angle of 135° is drawn here:
Hither, 45° is the reference angle of 135°.
Rules for Reference Angles in Each Quadrant
If the angle is in radians, then we do the same rules as for degrees by replacing 180° with π and 360° with 2π.
Example: Find the reference angle of 120°.
Solution: The given angle is, θ = 120°. We recognize that 120° lies in quadrant II. Applying the above rules, its reference angle is,
180 – θ = 180 – 120 = 60°
Therefore, the reference angle of 120° is 60°.
How to Find Reference Angles?
In the preceding section, we learned that we could find the reference angles using the set of rules mentioned in the table. That table runs only when the given angle lies between 0° and 360°. But what if the produced angle does not lie in this range? Let’s see how we can get the reference angles when the given angle is greater than 360°.
Steps to Find Reference Angles
The steps to obtain the reference angle of an angle are explained with an example. Let us see the reference angle of 480°.
Step 1: Obtain the coterminal angle of the given angle that lies between 0° and 360°.
The coterminal angle can be obtained either by adding or subtracting 360° from the given angle as many times as required. Let’s see the coterminal angle of 480° that lies between 0° and 360°. We will deduct 360° from 480° to find its coterminal angle.
480° – 360° = 120°
Step 2: If the angle from step 1 rests between 0° and 90°, then that angle itself is the reference angle of the given angle. If not, then we have to review whether it is closest to 180° or 360° and by how much.
Here, 120° does not lie within 0° and 90° and it is closest to 180° by 60°. i.e.,
180° – 120° = 60°
Step 3: The angle from step 2 is the reference angle of the provided angle.
Therefore, the reference angle of 480° is 60°.
This is how we can see reference angles of any given angle.
Important Notes:
- The reference angle of an angle is continuously non-negative i.e., a negative reference angle doesn’t exist.
- The reference angle of any angle always lies between 0 and π/2 (both inclusive).
Tricks to Find Reference Angles:
- We use the reference angle to find the values of trigonometric functions at an angle that is beyond 90°. For example, we can see that the coterminal angle and reference angle of 495° are 135° and 45° respectively.
sin 495° = sin 135° = +sin 45°.
We have added the + sign because 135° is in quadrant II, where sine is positive.
sin 495° = √2/2 [Using unit circle]
- If we use reference angles, we don’t require to remember the complete unit circle, instead we can just remember the first quadrant values of the unit circle.
FAQs
What is a Reference Angle?
A reference angle is an angle bounded within the terminal arm and the x-axis. It is a positive acute angle that lies within 0° to 90° or a 90-degree angle. It is necessary to understand the reference angle as it has its applications in finding the values of trigonometric ratios and in representing trigonometric functions on graphs.
How do you Find the Reference Angle?
To find the reference angle. let’s say of 500°, follow the steps given below:
- The first step is to find the coterminal angle of the given angle that lies between 0° to 360°. It is done by adding or subtracting 360° or 2π from the given angle as several times as required. So, in the case of 500°, if we subtract 360° from it, we will get 500° – 360° = 140°.
- The next step is to check whether the angle obtained in step 1 (140°) is closer to 180° or 360° and by how much. Here, 140° is closer to 180° by 40°.
- This angle is the reference angle of the presented angle. Therefore, 40° is the reference angle of 500°.
What is the Reference Angle for a 200° Angle?
Among the angles 180° and 360°, we can say that 200° is close to 180° by 20°. Thus, the reference angle of 200° is 20°.
Can Reference Angles be Negative?
A reference angle is a non-negative angle. It is continually positive and cannot be negative in measurement.
How to Find Reference Angle in Radians?
To see reference angles in radians is the same as finding them in degrees. The only variation is that in radians we replace 180° by π and 360° by 2π. Follow the rules given here to find reference angles in radians:
- Quadrant 1 – θ
- Quadrant 2 – π – θ
- Quadrant 3 – θ – π
- Quadrant 4 – 2π – θ